Find the slant height of a pyramid

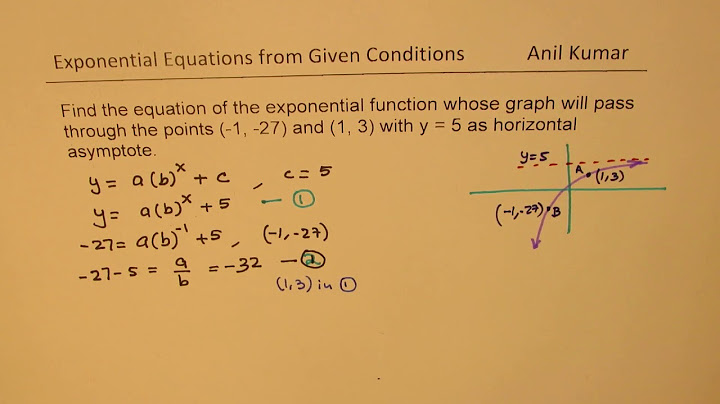
Video Transcript

Consider the net of a square pyramid with the shown dimensions. Find, to the nearest hundredth, the pyramid’s height. Find, to the nearest hundredth, the pyramid’s slant height.

Let’s begin this question by sketching what the pyramid would look like after it’s been created from the given net. So here we have our square pyramid. Because it’s a square, we know that all four sides of the base are equal in length. And from the diagram, we can see that this will be five centimeters. This length of four centimeters on the net of the pyramid represents one of the lateral side lengths on the pyramid, for example, here on the drawing of the pyramid.

This line drawn in pink on the pyramid is what we mean by the slant height. And that’s what we’ll need to calculate in the second part of this question.

So, let’s begin with the first part of this question, where we need to determine the height of the pyramid. The height of the pyramid will be the perpendicular height from the vertex to the center of the base. We can define the height as ℎ centimeters, and we observe that the height is not simply going to be one of the given measurements of four or five centimeters. In fact, to calculate the perpendicular height, we’ll need to create a triangle within the pyramid.

Let’s observe that we can draw the two lines here in pink to create the triangle. And since we know that the height is a perpendicular height, then we also know that this triangle will be a right triangle. We’re going to use this triangle as we already know that one of the edge lengths of the triangle is four centimeters. We can sketch this triangle in two dimensions. And we know that in the right triangle, it’s the hypotenuse which is four centimeters. And the other side length will be the height of the pyramid at ℎ centimeters.

We could therefore apply the Pythagorean theorem if we knew the length of this third side in the triangle. So, let’s see how we would determine the length of this third side. Returning to the net of the pyramid, we can see that this third side is the distance from the center of the square to one of its vertices. Let’s call this 𝑥 centimeters on each of our diagrams. This value of 𝑥 will be half the length of one of the diagonals of the square. Because this is a square, we know that it will have a 90-degree angle. So let’s apply the Pythagorean theorem to find the length of the diagonal.

Recall that the Pythagorean theorem states that the square of the hypotenuse is equal to the sum of the squares on the other two sides. Applying this within the square, we know that we have two shorter sides of five centimeters. If we define the length of the diagonal as 𝑦 centimeters, then plugging these values into the Pythagorean theorem, we would have five squared plus five squared equals 𝑦 squared. Since five squared is equal to 25, then we have simplified this to 50 is equal to 𝑦 squared. Taking the square root of both sides, we have that 𝑦 is equal to root 50 centimeters. But we can simplify this a little further. The square root of 50 can be simplified to the square root of 25 multiplied by the square root of two such that 𝑦 is equal to five root two centimeters.

Now that we have this value, we can determine that 𝑥 is half of this length. This is because the diagonals of a square bisect each other at right angles and the center point is at that intersection point of the diagonals. 𝑥 is therefore equal to one-half multiplied by five root two. More simply then, we can say that the length from the center of the square to the vertex is five over two root two centimeters. And remember that we calculated the value of 𝑥 so that we will have two known side lengths in this triangle to allow us to work out the value of ℎ. So, let’s clear some space for the next part of the workings.

In order to apply the Pythagorean theorem in this triangle, it can be helpful to note down the values of 𝑎, 𝑏, and 𝑐 that we’re using. It doesn’t matter which of the two shorter sides we define with 𝑎 and 𝑏, so let’s say that 𝑎 is ℎ centimeters, 𝑏 is five over two root two centimeters, and the hypotenuse 𝑐 is four centimeters. Plugging these into the Pythagorean theorem then, we have ℎ squared plus five over two root two squared is equal to four squared. And do be careful when we’re squaring this second term because we want to make sure that every value within that term is included in the squaring.

And if we aren’t using a calculator to square this second term, notice that we can square the five over two and square the root two separately to give us a value of 25 over two. On the right-hand side, four squared is 16. We can then subtract 25 over two from both sides of the equation, giving us ℎ squared is equal to seven over two. Taking the square root of both sides, we have that ℎ is equal to the square root of seven over two centimeters.

But since we were asked for the answer to the nearest hundredth, we need to find a decimal value for this answer. So we have that ℎ is equal to 1.8708 and so on centimeters. Rounding this to the nearest hundredth, we have that the height of the pyramid is approximately 1.87 centimeters to the nearest hundredth. And that’s the first part of this question answered.

Let’s clear some space and have a look at the second part of this question, where we need to calculate the slant height of the pyramid.

Recall that at the start of this question, we said that the line in pink would be the slant height of the pyramid. This would also appear here in the triangle within the net of the pyramid. The slant height will be a line from the top of the triangle at right angles to the base of the triangle. You might have already guessed that, once again, we’re going to apply the Pythagorean theorem.

If we define the slant height to be 𝑙 centimeters, then that means that we have one more length that we need to calculate in order to apply the Pythagorean theorem. This length at the base of the triangle will in fact be half the length of one side of the square. As the sides of the square are five centimeters each, then this length will be five over two centimeters, or 2.5 centimeters. When we are applying the Pythagorean theorem, the two shorter sides will be 𝑙 centimeters, that’s the slant height that we wish to calculate, and five over two centimeters. The hypotenuse will be four centimeters.

Substituting these into the Pythagorean theorem, we have 𝑙 squared plus five over two squared equals four squared. Evaluating the squares, we have 𝑙 squared plus 25 over four equals 16. Subtracting 25 over four from both sides of this equation, we have that 𝑙 squared is equal to 39 over four. Taking the square root of both sides of this equation, we have that 𝑙 is equal to the square root of 39 over four. And once again, we need to convert this into a decimal.

Using our calculators, we have that 𝑙 is equal to 3.1224 and so on centimeters. And rounded to the nearest hundredth, it’s 3.12 centimeters.

We have therefore answered both parts of this question. The pyramid’s height is approximately 1.87 centimeters, and the pyramid’s slant height is 3.12 centimeters.

It’s always worth checking that the answers that we give are sensible in terms of the given dimensions. And as we had a pyramid with dimensions of five centimeters and four centimeters, then both of our given answers would seem appropriate.

What is the formula for slant height of a pyramid?